Progress in quantum teleportation
量子传送的进展

Human beings have long dreamt of being able to transport themselves, or objects, from one location to another without actually travelling in between. A technology achieving this dream was imagined in science fiction stories and was called ‘teleportation’. Three decades ago, a similar idea appeared in quantum information and was dubbed quantum teleportation. Quantum teleportation enables the reconstruction of an arbitrary unknown quantum state of a real particle at a different location without actually transmitting the particle. Unlike fiction, quantum teleportation has physical constraints: it requires entanglement¹ between the two locations and the transmission of classical information, which is limited by the speed of light.
人类长期以来一直梦想能够将自己或物体从一个地方运输到另一个地方，而无需实际在两者之间旅行。实现这一梦想的技术在科幻故事中被设想，并被称为“瞬移”。三十年前，一个类似的想法出现在量子信息领域，并被称为量子瞬移。量子瞬移使得在不同位置重构真实粒子的任意未知量子态成为可能，而无需实际传输该粒子。与虚构不同，量子瞬移有物理限制：它需要两个位置之间的纠缠，并且需要传输经典信息，而这受到光速的限制。

Quantum teleportation was first proposed² in 1993 and in the early days, it was a theoretical scenario used in developing a formal theory of quantum information. But ever since, it has become an important concept in the now-established field of quantum information science³ and in quantum technologies, for example, in urban-scale quantum communication networks^4,5,6 or noisy intermediate-scale quantum computing^7,8,9. The essential feature of quantum teleportation, that of enabling the nonlocal transfer unknown information, can be used to overcome the distance limitation in quantum communication and the difficulty in realizing long-range interactions in quantum computing.
量子传送最早在 1993 年被提出，最初是一个用于发展量子信息正式理论的理论场景。但自那时以来，它已成为现已建立的量子信息科学领域和量子技术中的一个重要概念，例如在城市规模的量子通信网络或噪声中间规模的量子计算中。量子传送的基本特征是能够实现未知信息的非局部传输，这可以用来克服量子通信中的距离限制以及在量子计算中实现长距离交互的困难。

Quantum teleportation was first demonstrated experimentally^10,11 in 1997. Since then, scientists have performed quantum teleportation experiments in different quantum systems³ including photonic qubits (polarization and time bins), optical modes, NMR, atomic ensembles, trapped atoms and solid-state systems. These state-of-the-art experiments on quantum teleportation have been summarized elsewhere^3,12.
量子传送首次在 1997 年进行了实验演示。此后，科学家们在不同的量子系统中进行了量子传送实验，包括光子量子比特（偏振和时间窗）、光学模式、核磁共振、原子集群、被捕获的原子和固态系统。这些关于量子传送的最先进实验已在其他地方进行了总结。

Over the past 8 years, two main trends have emerged in the development of quantum teleportation (Fig. 1) owing to the increasing control over large-scale quantum systems. First, there is great interest in teleporting complex quantum states such as photons with information encoded in high dimensions^13,14 or in multiple degrees of freedom (DoFs)¹⁵, marking an important step towards the ultimate goal of teleporting all the information of a quantum system (Fig. 1a,d). Second, quantum teleportation has moved from the proof-of-principle demonstrations to practical applications. Great progress has been achieved in constructing long-distance quantum communication networks using remote quantum teleportation based on near-Earth satellites¹⁶ and metropolitan optical fibre networks^17,18 (Fig. 1b,e). Researchers have also laid the foundation for distributed quantum computing^19,20,21 by demonstrating quantum teleportation of controlled gates in superconducting qubits²², trapped ions²³ and single neutral-atom quantum electrodynamics (QED) cavities²⁴ (Fig. 1c,f).
在过去的 8 年中，由于对大规模量子系统的控制能力不断增强，量子传送的发展出现了两个主要趋势（图 1）。首先，对传送复杂量子态的兴趣日益浓厚，例如具有高维信息编码的光子 13,14 或多个自由度（DoFs）15，这标志着朝着传送量子系统所有信息的最终目标迈出了重要一步（图 1a,d）。其次，量子传送已从原理验证演示转向实际应用。在基于近地卫星 16 和大都市光纤网络 17,18 的远程量子传送中，构建长距离量子通信网络取得了重大进展（图 1b,e）。研究人员还通过在超导量子比特 22、被捕获的离子 23 和单中性原子量子电动力学（QED）腔 24 中演示受控门的量子传送，为分布式量子计算 19,20,21 奠定了基础（图 1c,f）。

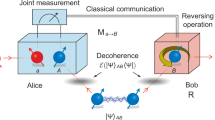
a, Quantum-state teleportation²: previous shared entanglement, Bell-state measurement (BSM), classical communication and unitary operations are used to transmit unknown quantum states without transmitting the particles themselves. The red, yellow and blue circles represent particles in the quantum teleportation protocol. S^A, S^B, S^C represent the quantum channels for transmitting particles A, B and C. $|{\psi }_A⟩$ is the input quantum state. $|{\psi }_B⟩$ is the output quantum state. An Einstein–Podolsky–Rosen (EPR) pair is a maximally entangled quantum state. b, Quantum entanglement swapping⁵: individuals in two pairs of independently entangled particles become entangled with two non-neighbouring particles through intermediate BSM. $|{\psi }_{AB}^{-}⟩$ and $|{\psi }_{CD}^{-}⟩$ are maximum entangled states. c, Quantum gate teleportation³²: through entanglement and classical channels, quantum-controlled operations between particles 1 and 2 (C_1,2) and particles 3 and 4 (C_3,4) are transferred to controlled operations between non-neighbouring particles 1 and 4. M2 and M3 represent projection measurements of 2 and 3 particles, respectively. d, Applications of quantum teleportation in quantum communication: ultra-long-distance quantum-state distribution can be realized through continuous quantum entanglement-swapping operations. e, Applications of quantum gate teleportation in quantum computing: quantum gate teleportation is important for distributed quantum computing to establish a connection between quantum computing nodes. Panel d is reproduced with permission from ref. ¹⁹⁴, Springer Nature Ltd. Panel e is reproduced with permission from ref. ⁹, Springer Nature Ltd.

Full size image

In this Review, we discuss the progress in quantum teleportation since 2015 and discuss the remaining challenges and future opportunities. We begin by introducing the theoretical progress, focusing on the new understanding of the nonclassical nature of quantum teleportation^25,26 and some potential applications based on quantum teleportation. Next, we overview quantum teleportation of complex quantum states, focusing on multiple DoFs¹⁵ and high-dimensional^13,14 systems. We then outline the development of teleportation in quantum communication^16,17,18,27 and quantum computing^22,23,24,28. Finally, we highlight future challenges and opportunities for quantum teleportation, hoping that ideas from recent experiments will provide new inspiration for the rapid development of quantum technologies.
在本综述中，我们讨论了自 2015 年以来量子传送的进展，并探讨了剩余的挑战和未来的机遇。我们首先介绍理论进展，重点关注对量子传送非经典性质的新理解以及基于量子传送的一些潜在应用。接下来，我们概述了复杂量子态的量子传送，重点关注多个自由度和高维系统。然后，我们概述了量子通信和量子计算中传送的发展。最后，我们强调了量子传送的未来挑战和机遇，希望近期实验中的想法能为量子技术的快速发展提供新的灵感。

In quantum teleportation, an unknown quantum state is transmitted from one location, usually referred to as the sender, Alice to another, the receiver, Bob. It is necessary to establish two channels between Alice and Bob, a quantum channel and a classical channel. In Fig. 1a, we take the simplest qubit system as an example to introduce the basic protocol of quantum teleportation². The quantum channel consists of an entangled pair shared between Alice and Bob,
在量子传输中，一个未知的量子态从一个位置（通常称为发送者，爱丽丝）传输到另一个位置（接收者，鲍勃）。有必要在爱丽丝和鲍勃之间建立两个通道，一个量子通道和一个经典通道。在图 1a 中，我们以最简单的量子比特系统为例，介绍量子传输的基本协议。量子通道由爱丽丝和鲍勃共享的纠缠对组成。

which is one of the four maximally entangled Bell states $(|{\mathrm{\Psi }}^{\pm }⟩=\frac{1}{\sqrt{2}}(|0⟩|1⟩\pm |1⟩|0⟩)$ and $(|{\mathrm{\Phi }}^{\pm }⟩=\frac{1}{\sqrt{2}}(|0⟩|0⟩\pm |1⟩|1⟩)$. Alice and Bob share this entangled state, in which particle 2 is with Alice and particle 3 is with Bob. Another party, called Charlie, provides the input particle 1 to be teleported to Bob in a general quantum state

in which α and β are complex coefficients that satisfy |α|² + |β|² = 1, unknown to both Alice and Bob. Then Alice performs a Bell-state measurement (BSM)^29,30,31 and randomly projects particles 1 and 2 each to one of the four Bell states with equal probability. Finally, Alice informs Bob of the BSM result through the classical channel, and Bob performs the corresponding Pauli or combinations of operators {I, X, Z, ZX} on his particle according to the results to recover the unknown quantum state $|{\mathrm{\Psi }}_A⟩$ of the particle of Charlie.

As an important protocol in quantum information, a basic framework for quantum teleportation was established and then many variants have been developed, such as quantum entanglement swapping⁵, gate teleportation³², port-based teleportation³³ and quantum teleportation networks^34,35. Recent theoretical advances in quantum teleportation focus on understanding its nonclassical nature and its potential applications.
作为量子信息中的一个重要协议，量子传送的基本框架已建立，随后发展了许多变体，如量子纠缠交换、门传送、基于端口的传送和量子传送网络。最近在量子传送方面的理论进展集中在理解其非经典特性及其潜在应用。

Nonclassical teleportation
非经典传送

Quantum entanglement is key to realizing lossless transmission of unknown quantum states in quantum teleportation. In the ideal teleportation scheme, the fidelity between the input and output states is F_tel = 1, but in real experiments one always obtains a smaller value. It is possible to simulate quantum teleportation using only classical correlations or classical measure–prepare strategies³⁶. This method, called classical teleportation, does not require the distribution of entangled states in advance, but it cannot achieve quantum teleportation with a theoretical fidelity of 1. The most commonly used method to verify nonclassical quantum teleportation is to construct fidelity-based quantum witness. If the fidelity of the transmitted quantum states can exceed the maximum fidelity that can be achieved by classical teleportation (F_cl), then the process is called nonclassical teleportation.
量子纠缠是实现量子传送中未知量子态无损传输的关键。在理想的传送方案中，输入态和输出态之间的保真度为 Ftel = 1，但在实际实验中，总是会得到一个较小的值。可以仅使用经典相关性或经典测量-准备策略来模拟量子传送。这种方法称为经典传送，不需要提前分发纠缠态，但无法实现理论保真度为 1 的量子传送。验证非经典量子传送的最常用方法是构建基于保真度的量子见证。如果传输的量子态的保真度超过经典传送所能达到的最大保真度（Fcl），则该过程称为非经典传送。

For the single-qubit case, the fidelity bound of classical teleportation²⁵ is F_cl = 2/3. This fidelity bound was later extended to an unknown state in a d-dimensional single-qudit case^37,38:
对于单量子比特的情况，经典传送的保真度界限为 Fcl = 2/3。这个保真度界限后来被扩展到 d 维单量子位的未知状态。

According to the fidelity criterion, not all entangled states can be used for nonclassical teleportation. However, a new benchmark has been proposed in recent years, which uses all the information available in the teleportation experiment and proves that all entangled states can achieve nonclassical quantum teleportation^26,39,40,41. The underlying idea of this approach is to carefully consider all the information in quantum teleportation, such as the density matrix of the input and output states, to show more nonclassical properties of quantum teleportation⁴².
根据保真度标准，并非所有纠缠态都可以用于非经典传输。然而，近年来提出了一种新的基准，它利用传输实验中所有可用的信息，并证明所有纠缠态都可以实现非经典量子传输。该方法的基本思想是仔细考虑量子传输中的所有信息，例如输入和输出态的密度矩阵，以展示量子传输的更多非经典特性。

Port-based teleportation is an important variant of quantum teleportation^3,33, which does not require any corrections on the side of the receiver (Bob). However, the disadvantage of this scheme is that the fidelity of teleportation converges to unity only when an infinite number of Bell pairs are shared. In the qubit case, in fact, it has been demonstrated that it impossible to realize nonclassical quantum teleportation when sharing one or two Bell pairs. Only when more than three Bell pairs are used, this scheme can achieve an average fidelity higher than two-thirds (reviewed elsewhere³³). In recent years, port-based teleportation has been widely expanded, for example, to improve its efficiency^43,44,45 and to propose high-dimensional⁴⁶ and continuous variable (CV) port-based teleportation⁴⁷. With the development of multicopy entanglement technology^48,49 and joint measurements^50,51, the first experimental demonstration of port-based teleportation can be realized.
基于端口的瞬时传输是量子瞬时传输的重要变体，它不需要接收方（鲍勃）进行任何纠正。然而，该方案的缺点是，只有在共享无限数量的贝尔对时，瞬时传输的保真度才会收敛到 1。在量子比特的情况下，实际上已经证明，当共享一个或两个贝尔对时，无法实现非经典的量子瞬时传输。只有在使用超过三个贝尔对时，该方案才能实现高于三分之二的平均保真度（在其他地方有综述）。近年来，基于端口的瞬时传输得到了广泛扩展，例如，提高其效率和提出高维和连续变量（CV）基于端口的瞬时传输。随着多副纠缠技术和联合测量的发展，基于端口的瞬时传输的首次实验演示可以实现。

Quantum teleportation-based theoretical applications
基于量子传送的理论应用

Quantum teleportation can not only be applied to practical tasks such as quantum communication or quantum computing but also can be used as an important theoretical tool in the theory of quantum information.
量子隐形传态不仅可以应用于量子通信或量子计算等实际任务，还可以作为量子信息理论中的一个重要理论工具。

Quantum channel simulation⁵² has been used to design a general and dimension-independent technique that reduces adaptive protocols into a block form⁵³. It simplifies the mathematical expression of complex adaptive protocols (for example, local operations and classical communication). This technique is called ‘teleportation stretching’ and is especially powerful when certain protocols are implemented over teleportation-covariant channels⁵³ (such as Pauli, erasure⁵⁴ or Gaussian channels⁵⁵). With this new tool, scientists established the ultimate capacity limits of CV and discrete-variable (DV) quantum key distribution in point-to-point lossy^53,56, multipoint⁵⁷, repeater-assisted^58,59 and network⁶⁰ quantum communications. Owing to its capability to simplify adaptivity, teleportation stretching is used to achieve the ultimate precision of adaptive noise estimation for any teleportation-covariant channel, thus extending the teleportation channel simulation technique to quantum metrology^61,62,63 and quantum channel discrimination^64,65. Note that port-based teleportation is also an important tool in the theory of quantum information, for example, in the optimization^66,67 and resource quantification^68,69 of programmable quantum processors, and for the exploration of the fundamental limits of quantum channel discrimination^64,65.
量子信道模拟 52 已被用于设计一种通用且与维度无关的技术，将自适应协议简化为块形式 53。这简化了复杂自适应协议的数学表达（例如，局部操作和经典通信）。该技术称为“传送拉伸”，在某些协议通过传送协变信道 53（如保利信道、擦除信道 54 或高斯信道 55）实施时尤其强大。借助这一新工具，科学家们建立了点对点有损 53,56、多点 57、辅助中继 58,59 和网络 60 量子通信中 CV 和离散变量（DV）量子密钥分发的最终容量极限。由于其简化自适应性的能力，传送拉伸被用于实现任何传送协变信道的自适应噪声估计的最终精度，从而将传送信道模拟技术扩展到量子计量学 61,62,63 和量子信道区分 64,65。 请注意，基于端口的瞬移在量子信息理论中也是一个重要工具，例如，在可编程量子处理器的优化 66,67 和资源量化 68,69 中，以及在量子信道区分的基本限制探索 64,65 中。

Quantum teleportation also has a role in understanding many physical phenomena such as quantum catalysis⁷⁰, quantum decoherence^71,72, directly measuring quantum wave function⁷³, macroscopic quantum⁷⁴, the indistinguishability of photons^75,76 and so on.
量子传输在理解许多物理现象方面也发挥着作用，例如量子催化 70、量子退相干 71,72、直接测量量子波函数 73、宏观量子 74、光子的不可区分性 75,76 等。

Photonic systems have various DoFs such as polarization⁷⁷, orbital angular momentum (OAM)⁷⁸, time bins⁷⁹, path⁸⁰ and frequency⁸¹, most of which can encode in high dimensions. In the real world, a single particle often contains many DoFs, forming a complex quantum state. BSM is the key technology of qubit and complex quantum-states teleportation. In the qubit case, owing to the limit of linear optics, the BSM success probability is at most 50%^82,83. This limitation can be overcome by using the entanglement in multiple DoFs (hyper-entanglement)^84,85,86 or nonlinear processes⁸⁷. Theoretical studies prove that this can also be achieved by adding auxiliary photon pairs^88,89 or error correction codes^90,91. With the help of auxiliary photons, BSM with a success probability of 57.9% was reported in an experiment⁹². In the case of complex quantum states, the implementation of BSM is more difficult. In this section, we discuss quantum teleportation of photonic complex quantum states, including multiple DoFs quantum states, high-dimensional quantum-states and hybrid systems, the ultimate goal being to teleport all the information of a quantum system.
光子系统具有多种自由度，如偏振 77、轨道角动量（OAM）78、时间窗 79、路径 80 和频率 81，其中大多数可以在高维中编码。在现实世界中，单个粒子通常包含许多自由度，形成复杂的量子态。贝尔态测量（BSM）是量子比特和复杂量子态传输的关键技术。在量子比特的情况下，由于线性光学的限制，BSM 的成功概率至多为 50%82,83。通过利用多自由度中的纠缠（超纠缠）84,85,86 或非线性过程 87，可以克服这一限制。理论研究证明，通过添加辅助光子对 88,89 或错误纠正码 90,91，也可以实现这一点。在实验中，借助辅助光子，报告了成功概率为 57.9%的 BSM92。在复杂量子态的情况下，实施 BSM 更为困难。在本节中，我们讨论光子复杂量子态的量子传输，包括多自由度量子态、高维量子态和混合系统，最终目标是传输量子系统的所有信息。

Quantum states in multiple degrees of freedom
多自由度下的量子态

A single quantum particle can naturally have different internal and external DoFs with coherent coupling between them. Multiple DoFs represent more operational possibilities and can be used to implement more transmission protocols such as superdense quantum teleportation^93,94 and quantum teleportation from polarization to OAM DoFs⁹⁵. A basic challenge is to teleport multiple DoFs at the same time, which is necessary for a complete description of quantum particles.
单个量子粒子可以自然地具有不同的内部和外部自由度，并且它们之间存在相干耦合。多个自由度代表更多的操作可能性，可以用于实现更多的传输协议，例如超密集量子隐形传态和从偏振到轨道角动量自由度的量子隐形传态。一个基本挑战是同时隐形传态多个自由度，这对于对量子粒子的完整描述是必要的。

Achieving multi-DoF quantum teleportation requires sharing hyper-entanglement in advance and implementing BSM on multiple DoFs. In photonic systems, the most difficult task is to implement hyper-BSM because it requires coherent controlled gates between independent qubits of different DoFs and measuring one of the DoFs without interfering with others⁹⁶. In 2007, it was theoretically proved that it is impossible to distinguish hyper-entangled Bell states deterministically using only linear optics without auxiliary particles⁹⁷ because BSMs with different DoFs will interfere with each other, leading to failure of simultaneous recognition of all hyper-entangled Bell states. However, some protocols have been proposed for quantum teleportation with multiple DoFs^98,99 and in 2015, scientists successfully overcame this challenge and simultaneously teleported photonic polarization and OAM DoFs for the first time¹⁵ (Fig. 2a). This was possible owing to the creative use of quantum non-demolition measurement (QND), which refers to the observation of a single photon without destroying it and preserving its quantum information intact. Interestingly, quantum teleportation assisted by entanglement can achieve probabilistic QND detection. The protocol in ref. ¹⁵ can also be extended to the quantum teleportation of more DoFs at the same time.
实现多自由度量子传送需要提前共享超纠缠并在多个自由度上实施贝尔态测量（BSM）。在光子系统中，最困难的任务是实现超贝尔态测量，因为这需要在不同自由度的独立量子比特之间实现相干控制门，并在不干扰其他自由度的情况下测量其中一个自由度。2007 年，理论上证明仅使用线性光学而不借助辅助粒子无法确定性地区分超纠缠贝尔态，因为不同自由度的贝尔态测量会相互干扰，导致无法同时识别所有超纠缠贝尔态。然而，已经提出了一些多自由度量子传送的协议，并且在 2015 年，科学家们首次成功克服了这一挑战，同时传送了光子的极化和轨道角动量（OAM）自由度。这得益于量子非破坏性测量（QND）的创造性应用，该方法指的是在不破坏单个光子的情况下观察它，并保持其量子信息的完整性。 有趣的是，受纠缠辅助的量子传送可以实现概率性 QND 检测。参考文献 15 中的协议也可以扩展到同时传送更多自由度。

a, Concept for quantum teleportation with multiple degrees of freedom (DoFs)¹⁵. The polarization and orbital angular momentum (OAM) DoFs of a photon are teleported simultaneously. To avoid the influence of the Bell-state measurement (BSM) of the polarization DoF (in PBS) on the OAM DoF (in BS), the experimenter used a quantum non-demolition measurement (QND), which is based on assisted entanglement quantum teleportation. The success probability of the final multiple-DoF BSM is 1/32. U^O and U^S represent the unitary operations on OAM and spin DoFs, respectively. b, Concept for quantum teleportation of a 3D path state. To realize the path DoF of the 3D BSM, a pair of entangled photons is used to assist in ref. ¹³ (as illustrated here), and a single-photon-assisted Fourier transform with three inputs and three outputs is used in ref. ¹⁴. In these two experiments, the success probabilities of the 3D BSM are 1/162 and 1/81, respectively. c, Concept for high-dimensional OAM^124,125 and Hermite-Gaussian-mode¹²⁵ quantum teleportation. The experimenter prepared the spatial-mode entangled state through SPDC. HDBSM can be achieved using mode conservation in the up-conversion of a nonlinear crystal (NLC). APD, avalanche photodiode detector; BS, beam splitter; h-BSM, hybrid Bell-state measurement; HDBSM, high-dimensional Bell-state measurement; PBS, polarization beam splitter; SLM, spatial light modulator; SPDC, spontaneous parametric down-conversion; U³, 3D unitary operation. Panel a is reproduced with permission from ref. ¹⁵, Springer Nature Ltd; panel b is reproduced with permission from ref. ¹³, APS; panel c, image courtesy of Berenice Sephton.
量子传送的多自由度（DoFs）概念 15。光子的偏振和轨道角动量（OAM）自由度同时被传送。为了避免偏振自由度（在 PBS）的贝尔态测量（BSM）对 OAM 自由度（在 BS）产生影响，实验者使用了基于辅助纠缠量子传送的量子非破坏测量（QND）。最终多自由度 BSM 的成功概率为 1/32。UO 和 US 分别表示对 OAM 和自旋自由度的单位操作。b，三维路径态的量子传送概念。为了实现三维 BSM 的路径自由度，使用一对纠缠光子来辅助参考文献 13（如这里所示），并在参考文献 14 中使用了具有三个输入和三个输出的单光子辅助傅里叶变换。在这两个实验中，三维 BSM 的成功概率分别为 1/162 和 1/81。c，高维 OAM124,125 和 Hermite-Gaussian 模式 125 量子传送的概念。实验者通过自发参量下转换（SPDC）准备了空间模式纠缠态。 HDBSM 可以通过非线性晶体（NLC）的上转换中的模式守恒来实现。APD，雪崩光电二极管探测器；BS，光束分 splitter；h-BSM，混合贝尔态测量；HDBSM，高维贝尔态测量；PBS，偏振光束分 splitter；SLM，空间光调制器；SPDC，自发参数下转换；U3，三维单位操作。面板 a 经 Springer Nature Ltd.许可转载自参考文献 15；面板 b 经 APS 许可转载自参考文献 13；面板 c，图片由 Berenice Sephton 提供。

Full size image

Researchers have teleported five hyper-quantum states with a fidelity greater than the classical upper bound of 0.4 for simultaneous quantum teleportation of two DoFs state in one photon^37,38. The last teleported quantum state was a hyper-entangled state, which is an entangled state formed by polarization and OAM DoF on the same photon and its fidelity exceeded the bound of the entangled state (0.5)¹⁰⁰. Because of the existence of auxiliary particles, the whole quantum teleportation was a six-photon experiment, and the photon counting rate was approximately 15 clicks per hour.
研究人员已经成功传送了五个超量子态，其保真度超过了经典上限 0.4，实现在一个光子中同时量子传送两个自由度状态。最后传送的量子态是一个超纠缠态，这是由同一光子的偏振和轨道角动量自由度形成的纠缠态，其保真度超过了纠缠态的界限（0.5）。由于存在辅助粒子，整个量子传送实验是一个六光子实验，光子计数率约为每小时 15 次点击。

Photon hyper-entanglement has been realized with two or more DoFs^{101,102,103,104} and can be distributed over an 11-km fibre channel¹⁰⁵ and a 1.2-km free-space channel¹⁰⁶. When this is combined with QND measurement, one can expect that long-distance quantum teleportation of more DoFs will be achieved in the future.
光子超纠缠已经在两个或更多自由度（DoFs）中实现，并可以通过 11 公里的光纤通道和 1.2 公里的自由空间通道进行分发。当这与量子非破坏性测量（QND）相结合时，可以预期未来将实现更多自由度的长距离量子传送。

Quantum states in high dimensions
高维量子态

Compared with two-level systems (qubits), high-dimensional systems (multilevel systems encoding qudits) are more prevalent in nature. The ability to coherently control high-dimensional quantum states is important for developing advanced quantum technologies^{80,81,107,108,109}. Compared with conventional qubit systems, qudit systems can offer extended possibilities such as both higher capacity^110,111,112 and more noise resilience in quantum communications^113,114,115, more efficient quantum simulation¹¹⁶ and computation¹¹⁷ and greater violation of Bell inequalities¹¹⁸.
与二级系统（量子比特）相比，高维系统（编码量子位的多级系统）在自然界中更为普遍。相干控制高维量子态的能力对于开发先进的量子技术至关重要。与传统的量子比特系统相比，量子位系统可以提供更广泛的可能性，例如在量子通信中具有更高的容量和更强的抗噪声能力，更高效的量子模拟和计算，以及更大的贝尔不等式违反。

The challenges in high-dimensional quantum teleportation are: preparing high-quality high-dimensional entanglement sources and implementing high-dimensional BSMs¹². Progress has been made in developing high-dimensional entanglement preparation technologies that use OAM⁷⁸, time bins⁷⁹, paths^80,119 and frequency⁸¹. Achieving high-dimensional BSM has become urgent for realizing high-dimensional quantum teleportation.
高维量子传输中的挑战包括：准备高质量的高维纠缠源和实现高维贝尔态测量（BSM）。在利用轨道角动量（OAM）、时间窗、路径和频率等技术开发高维纠缠制备技术方面已经取得了一定进展。实现高维贝尔态测量对于实现高维量子传输变得迫在眉睫。

In 2002, it was pointed out that partial high-dimensional BSM requires additional auxiliary particles¹²⁰. The key insight is that it is impossible to clearly distinguish a single Bell state from other d² − 1 states using only linear optics, and all Bell states can only be divided into several categories^97,111. Similar to the concept of genuine multipartite entanglement, genuine high-dimensional teleportation cannot be simulated by low-dimensional quantum teleportation with a fidelity exceeding (d − 1)/d. In 2017, experimenters successfully demonstrated the teleportation of multiple OAM states¹²¹, which makes the simultaneous transmission of 2D subspaces possible with high-dimensional quantum states.
在 2002 年，有人指出部分高维 BSM 需要额外的辅助粒子。关键的见解是，仅使用线性光学无法清晰地区分单一的贝尔态与其他 d² - 1 态，所有贝尔态只能被划分为几类。与真正的多方纠缠概念类似，真正的高维传输无法通过低维量子传输模拟，且保真度超过(d - 1)/d。在 2017 年，实验者成功演示了多个 OAM 态的传输，这使得高维量子态的 2D 子空间的同时传输成为可能。

In 2019, genuine 3D quantum teleportation was realized in two experiments^13,14 using the path DoF of photons (Fig. 2b). The difference between these two experiments is that the two research groups used different high-dimensional BSM schemes. In one experiment, a Fourier transformation with three inputs and three outputs was used, which requires an auxiliary particle¹⁴. This method can be extended to any number of dimensions, requiring (d − 2) auxiliary photons. This experiment requires a pair of 3D entangled photons, a transmitted photon and an auxiliary photon, finally resulting in a four-photon experiment. Twelve states from four mutually unbiased bases were transmitted¹²² with an average fidelity of 75 ± 1%, which exceeds the classical limit (1/2) and the genuine 3D limit (2/3). The count rate of this experiment was 400 counts per hour.
在 2019 年，真正的 3D 量子传送在两个实验中实现了，使用了光子的路径自由度（图 2b）。这两个实验之间的区别在于两个研究小组使用了不同的高维贝尔态测量方案。在一个实验中，使用了一个具有三个输入和三个输出的傅里叶变换，这需要一个辅助粒子。该方法可以扩展到任意维度，需用到(d - 2)个辅助光子。该实验需要一对 3D 纠缠光子，一个传输光子和一个辅助光子，最终形成一个四光子实验。来自四个相互无偏基的十二个态以 75 ± 1%的平均保真度被传输，这超过了经典极限（1/2）和真正的 3D 极限（2/3）。该实验的计数率为每小时 400 次计数。

In the other experiment, a pair of polarization-entangled states was used as an auxiliary in the 3D BSM¹³ (Fig. 2b). This method can also be extended to any number of dimensions, requiring (log 2(d) − 1) entangled pairs. Nine information-complete states were teleported, and the process matrix of quantum teleportation was reconstructed with a fidelity of F = 59.6 ± 3.7%. Genuine high-dimensional quantum teleportation was verified to have been achieved by calculating the non-simulability of the process matrix from 2D quantum teleportation²⁶. Because entangled-photon pairs were used as auxiliary particles, the final experiment was a six-photon experiment with a count rate of 10 counts per hour.
在另一个实验中，使用了一对偏振纠缠态作为 3D BSM13 的辅助（图 2b）。该方法也可以扩展到任意维度，所需的纠缠对数量为(log 2(d) − 1)。九个信息完整态被传送，量子传送的过程矩阵以 F = 59.6 ± 3.7%的保真度被重构。通过计算来自 2D 量子传送的过程矩阵的不可模拟性，验证了真正的高维量子传送已被实现。由于使用了纠缠光子对作为辅助粒子，最终实验是一个六光子实验，计数率为每小时 10 次计数。

The limitation of linear optics in BSM is mainly due to the fact that photons do not interact with each other, but this can be overcome by using a nonlinear photon process. In 2000, nonlinear processes were successfully used for simultaneous deterministic measurement of four polarized Bell states⁸⁷. In 2022, two experiments used a nonlinear process to teleport a high-dimensional photonic quantum state, with one experiment encoding the state in OAM and the other encoding it in Hermite-Gaussian (HG) modes. The core principle of using nonlinearity for high-dimensional BSM is that two photons in different high-dimensional Bell states acquire distinguishable single-photon states in the up-conversion process¹²³ (Fig. 2c).
在贝尔态测量（BSM）中，线性光学的局限性主要是由于光子之间不相互作用，但这可以通过使用非线性光子过程来克服。2000 年，非线性过程成功用于同时确定性测量四个极化贝尔态。在 2022 年，两个实验使用非线性过程传送高维光子量子态，其中一个实验将态编码在轨道角动量（OAM）中，另一个实验则将其编码在厄米-伽乌斯（HG）模式中。利用非线性进行高维贝尔态测量的核心原理是，在上转换过程中，处于不同高维贝尔态的两个光子获得可区分的单光子态。

In an experiment¹²⁴ using 3D OAM BSM, the average quantum teleportation fidelity of 12 mutually unbiased base quantum states was F = 0.879, which proves that it was nonclassical and a genuine high-dimensional process. Another experiment¹²⁵ (Fig. 2c) achieved high-dimensional quantum teleportation with 9D HG modes in addition to OAM. The fidelity was 99 ± 3% for 4D OAM states and 81 ± 2% for 9D HG mode states. Although these two experiments used a coherent source, with future advances in nonlinear optics they could also be performed at single-photon level without changing the single-entangled-pair configuration.
在一项使用 3D OAM BSM 的实验中，12 个相互无偏基量子态的平均量子传输保真度为 F = 0.879，这证明了它是非经典的，并且是一个真正的高维过程。另一项实验（图 2c）在 OAM 的基础上实现了 9D HG 模式的高维量子传输。4D OAM 态的保真度为 99 ± 3%，9D HG 模式态的保真度为 81 ± 2%。尽管这两项实验使用了相干源，但随着非线性光学的未来进展，它们也可以在单光子水平上进行，而无需改变单纠缠对配置。

Note that an optical image has been successfully teleported by exploring the full transverse spatial entanglement¹²⁶. Combined with phase information, this technology is expected to realize quantum teleportation of spatial mode wave functions in the future¹²⁷.
请注意，通过探索完整的横向空间纠缠，光学图像已成功被传送。结合相位信息，这项技术预计将在未来实现空间模式波函数的量子传送。

The concepts and techniques of the experiments discussed in this section can be transferred to other high-dimensional DoFs. Combining them with high-dimensional entanglement distribution technology^{81,106,119,128,129} can achieve high-dimensional quantum teleportation over a long distance. With the development of high-dimensional quantum memory technologies^{130,131,132,133,134,135}, high-dimensional quantum teleportation will hopefully be used in high-dimensional quantum networks¹³⁶ to improve performances.
本节讨论的实验概念和技术可以转移到其他高维自由度。将它们与高维纠缠分发技术结合，可以实现长距离的高维量子传输。随着高维量子存储技术的发展，高维量子传输有望在高维量子网络中应用，以提高性能。

Quantum states in continuous variables and hybrid approaches
连续变量中的量子态与混合方法

Infinite-dimensional Hilbert spaces, which are CV systems^55,137, are important to optical quantum teleportation³. In the early years, CV quantum teleportation was demonstrated in optical modes¹³⁸ and collective spins of atomic ensembles^139,140.
无限维希尔伯特空间，作为连续变量系统，是光学量子传输的重要组成部分。在早期，连续变量量子传输在光学模式和原子集体自旋中得到了验证。

CV quantum teleportation is not only an important tool for Gaussian channel simulation⁵² but also to other theoretical studies. For example, a resource theory framework has been constructed to calculate the minimum entanglement consumption required for CV quantum teleportation^{56,141,142,143,144}. Furthermore, a concatenated error-correction method based on CV quantum teleportation has been used to build a loss-tolerant quantum repeater architecture¹⁴⁵. A sequential phase estimation protocol enabled by repeated CV teleportation has shown both super resolution and super sensitivity¹⁴⁶.
CV 量子隐形传态不仅是高斯信道模拟的重要工具 52，还对其他理论研究具有重要意义。例如，已经构建了一个资源理论框架，以计算进行 CV 量子隐形传态所需的最小纠缠消耗 56,141,142,143,144。此外，基于 CV 量子隐形传态的级联纠错方法已被用于构建一种容错量子中继架构 145。通过重复 CV 隐形传态实现的顺序相位估计协议显示了超分辨率和超灵敏性 146。

From the experimental point of view, efforts have been dedicated to increasing the capacity of quantum teleportation channels in CV systems. One group innovatively used a high-gain parametric amplifier based on four-wave mixing in an ⁸⁵Rb vapour cell to realize BSM without detection, which avoided the optic-electro and electro-optic conversion required by traditional BSM in a CV system⁵⁵. Using such all-optical feedforward technology, measurement-free all-optical quantum teleportation¹⁴⁷ and quantum entanglement swapping¹⁴⁸ protocols were experimentally realized, which are expected to release the bandwidth limitation of CV quantum information protocols in building broadband quantum networks. Multiplexing optical modes can effectively increase the transmission capacity of quantum teleportation. A CV system was successfully teleported using nine OAM¹⁴⁷ and five optical frequency comb¹⁴⁹ multiplexed channels.
从实验的角度来看，研究人员致力于提高连续变量系统中量子隐形传态通道的容量。一个研究小组创新性地使用基于四波混频的高增益参数放大器，在 85Rb 蒸汽池中实现了无检测的贝尔态测量，这避免了传统连续变量系统中贝尔态测量所需的光电和电光转换。利用这种全光前馈技术，实验上实现了无测量的全光量子隐形传态和量子纠缠交换协议，这有望释放构建宽带量子网络时连续变量量子信息协议的带宽限制。复用光模式可以有效增加量子隐形传态的传输容量。一个连续变量系统成功地使用九个轨道角动量和五个光频梳复用通道进行了隐形传态。

CV quantum information processing benefits from complete BSM, high detection efficiency and unambiguous state discrimination. However, its sensitivity to loss limits its fidelity. DV systems can effectively resist losses, but they are limited by probabilistic operations and BSM. Combining the two in a hybrid architecture could have significant advantages^150,151.
CV 量子信息处理得益于完整的贝尔态测量、高检测效率和明确的状态区分。然而，其对损失的敏感性限制了其保真度。DV 系统能够有效抵抗损失，但受到概率操作和贝尔态测量的限制。将两者结合在混合架构中可能具有显著优势。

Many attempts have been made to teleport hybrid quantum systems but the biggest challenge is preparing hybrid entanglement and special BSM. Hybrid entanglement of Fock-squeezed states¹⁵², cat-Fock states^153,154 and polarization-cat states¹⁵⁵ can be used to build hybrid quantum networks. When combined with other DV quantum states or entanglement types such as polarization entanglement and Fock-state entanglement, this can achieve teleportation of hybrid quantum states or hybrid quantum entanglement swapping.
许多尝试已经被提出以传送混合量子系统，但最大的挑战是准备混合纠缠和特殊的贝尔态测量（BSM）。福克压缩态的混合纠缠、猫-福克态和偏振猫态可以用于构建混合量子网络。当与其他离散变量（DV）量子态或纠缠类型（如偏振纠缠和福克态纠缠）结合时，这可以实现混合量子态的传送或混合量子纠缠的交换。

BSM is also a key factor in such experiments. For instance, using a cat-state BSM, the experimenter can teleport a CV cat qubit to a DV Fock qubit state¹⁵³. Although only one Bell state has been recognized in this experiment¹⁵³, in principle, four Bell states can be identified deterministically at the same time by including a detector that can distinguish the number of photons. Entanglement between a DV polarization qubit and CV opposite-amplitude coherent state has been realized through quantum entanglement swapping using a BSM of a polarization DoF¹⁵⁵. Heralded creation of a hybrid entrance has been realized at a 47-ns delay distance using a Fock-state BSM with a single-shot counter and a homodyne detector for hybrid entanglement swapping¹⁵⁴. These experiments open the prospect of connecting heterogeneous nodes in a network with increased integration and new functions.
BSM 也是此类实验中的一个关键因素。例如，使用猫态 BSM，实验者可以将 CV 猫量子比特传送到 DV Fock 量子比特状态。尽管在此实验中仅识别出一个贝尔态，但原则上，通过包括一个能够区分光子数量的探测器，可以同时确定四个贝尔态。通过使用偏振自由度的 BSM，已经实现了 DV 偏振量子比特与 CV 相反幅度相干态之间的纠缠交换。利用具有单次计数器和同相干探测器的 Fock 态 BSM，在 47 纳秒延迟距离下实现了混合入口的预示创建。这些实验为在网络中连接异构节点提供了更高的集成度和新功能的前景。

Quantum teleportation can be used to transmit an unknown quantum state without it actually travelling along the way, which is important in quantum communication⁴. The quantum non-cloning principle³⁶ that an unknown quantum state cannot be copied limits the transmission distance of quantum communication. For a direct fibre-based link from the transmitter to the receiver, the key rate decreases exponentially with the transmission distance and cannot surpass the Pirandola–Laurenza–Ottaviani–Banchibound −log₂(1 − η)⁵³, where η denotes the transmittance of the link. As a variant of quantum teleportation, quantum entanglement swapping (Fig. 1b) can be used to overcome this limit. The basic principle of quantum entanglement swapping is that the key rate attenuation can be reduced from exponential to polynomial through segmented communication. Quantum memories¹⁵⁶ can effectively improve the efficiency of quantum entanglement swapping among multiple nodes. Memory-based quantum entanglement swapping can be used in a quantum repeater⁵ and has been demonstrated in an atomic ensemble¹⁵⁷, a single atom^6,158, an ion trap¹⁵⁹, rare-earth solid state memory^160,161 and a nitrogen-vacancy (NV) centre¹⁶². However, these experiments have mainly focused on the platforms or on short distances. The progress in quantum repeaters has been discussed in other reviews^{163,164,165,166}. In recent years, interesting variations of teleportation have also emerged in quantum communication, such as controlled quantum teleportation^112,167 and teleportation of a shared quantum secret¹⁶⁸.
量子隐形传态可以用来传输未知的量子态，而不需要它实际沿途移动，这在量子通信中非常重要。量子不可克隆原理表明，未知的量子态无法被复制，这限制了量子通信的传输距离。对于从发射器到接收器的直接光纤连接，密钥速率随着传输距离的增加呈指数下降，无法超过 Pirandola–Laurenza–Ottaviani–Banchi 界限−log2(1 − η)，其中η表示链接的透射率。作为量子隐形传态的一种变体，量子纠缠交换（图 1b）可以用来克服这一限制。量子纠缠交换的基本原理是，通过分段通信，可以将密钥速率的衰减从指数级降低到多项式级。量子存储器可以有效提高多个节点之间量子纠缠交换的效率。 基于记忆的量子纠缠交换可以用于量子中继器，并已在原子集群、单个原子、离子阱、稀土固态存储器和氮空位（NV）中心中得到了验证。然而，这些实验主要集中在平台或短距离上。量子中继器的进展已在其他综述中讨论。近年来，量子通信中也出现了一些有趣的传送变体，如受控量子传送和共享量子秘密的传送。

We subsequently overview recent developments of long-distance quantum teleportation, including photonic quantum teleportation based on optical fibre networks, free-space satellites and integrated systems that are expected to be implemented on a large scale.
我们随后概述了远程量子传送的最新进展，包括基于光纤网络的光子量子传送、自由空间卫星以及预计将在大规模上实施的集成系统。

Optical fibre network 光纤网络

In a future quantum network^19,169, multiple nodes will share entanglement through quantum repeaters to form a quantum entanglement connection. Quantum networks with remote nodes require each node to have an independent quantum entanglement source, and photons from different sources are indistinguishable when quantum interference occurs after long-distance distribution¹⁷⁰.
在未来的量子网络中，多个节点将通过量子中继器共享纠缠，以形成量子纠缠连接。具有远程节点的量子网络要求每个节点拥有独立的量子纠缠源，并且在长距离分发后，当发生量子干涉时，来自不同源的光子是不可区分的。

Achieving high-visibility and highly stable interference between photons in a long-distance optical fibre network requires a high-precision synchronization system and a quantum channel stabilization system. However, in the real world, the effective length and polarization of the quantum channel will fluctuate, making the two photons distinguishable and reducing the interference visibility. This situation did not exist in the previous proof-of-concept local experiments in stable laboratory environments^171,172.
在长距离光纤网络中实现光子的高可见性和高度稳定的干涉需要高精度的同步系统和量子通道稳定系统。然而，在现实世界中，量子通道的有效长度和偏振会波动，使得两个光子可区分，从而降低干涉可见性。这种情况在之前的概念验证本地实验中并不存在，这些实验是在稳定的实验室环境中进行的。

In 2016, two groups independently realized long-distance quantum teleportation in metropolitan optical networks in the cities of Hefei and Calgary (Fig. 3a).
在 2016 年，两个团队独立地在合肥和卡尔加里城市的 metropolitan 光网络中实现了长距离量子传输（图 3a）。

a, Quantum teleportation was achieved in metropolitan optical networks in the cities of Calgary¹⁸ and Hefei¹⁷. The map shows the experiment in Calgary. The experimenters successfully synchronized two independent photon sources (in nodes A and C) with an interval of 8.2 km. Quantum teleportation was demonstrated in a three-node fibre-optic network with lengths of 6.2 km and 11.1 km. A, B and C represent the nodes of Alice, Bob and Charlie. b, Quantum teleportation was realized in free space from an observatory ground station in Ngari to the Micius satellite over a distance of 1,400 km. In the experiment¹⁶, 780-nm photons were used as the quantum communication carriers. In the right figure, the Ngari observation station is on the ground, and the Micius satellite is in the air, and the physical distance between the ground station and the satellite varied from a maximum of 1,400 km to a minimum of 500 km. As a result, the channel loss of the uplink varied from 52 dB to 41 dB. The four-photon source preparation and Bell measurement were completed at the Ngari observatory station. One photon of the entangled photon source was sent to the Micius satellite to establish a ground-to-satellite entanglement distribution. c, Quantum teleportation in an integrated chip²⁷. Four microring resonators were prepared on silicon to generate single photons with high spectral purity and high heralding efficiency by eliminating the requirement of spectral filtering. A connection was established using a 10-m single-mode fibre, enabling quantum teleportation from one chip to another. Subpanels on the right are microring resonator structures and teleportation receiver. HOM, Hong–Ou–Mandel effect; BSM, Bell-state measurement; CC, classical channels; QC, quantum channel; FBG, fibre Bragg grating; IM, intensity modulator; FM, Faraday mirror; MZI, Mach–Zehnder interferometer; BS, beam splitter; PBS, polarization beam splitter; HWP, half wave plate; LD, laser diode; PDs, photodiodes; PPLN, periodically poled lithium niobate; DM, dichroic mirror; FP, Fabry–Perot cavity; FPGA, field-programmable gate array; VEDL, variable electronic delay line; DWDM, dense-wavelength division multiplexers; AWG, arbitrary waveform generators; Si-APD, silicon avalanche photodiodes; SPD, single-photon detector; SNSPD, superconducting nanowire single-photon detectors; CMOS, camera; SHG, second harmonic generation; SPDC, spontaneous parametric down-conversion. Panel a is reproduced from ref. ¹⁸, Springer Nature Ltd; panel b is reproduced from ref. ¹⁶, Springer Nature Ltd; panel c is reproduced from ref. ²⁷, Springer Nature Ltd.
量子隐形传态在卡尔加里和合肥的城市光网络中实现。地图显示了卡尔加里的实验。实验者成功地将两个独立的光子源（在节点 A 和 C）同步，间隔为 8.2 公里。量子隐形传态在一个三节点光纤网络中演示，网络长度为 6.2 公里和 11.1 公里。A、B 和 C 分别代表爱丽丝、鲍勃和查理的节点。量子隐形传态在自由空间中实现，从那曲的地面观测站到墨子卫星，距离为 1400 公里。在实验中，使用 780 纳米的光子作为量子通信载体。在右侧图中，那曲观测站位于地面，墨子卫星在空中，地面站与卫星之间的物理距离从最大 1400 公里变化到最小 500 公里。因此，上行链路的信道损耗从 52 dB 变化到 41 dB。四光子源的制备和贝尔测量在那曲观测站完成。 一个纠缠光子源的光子被发送到墨子卫星，以建立地面到卫星的纠缠分发。c，集成芯片中的量子传送 27。四个微环谐振器在硅上制备，以通过消除光谱过滤的要求生成具有高光谱纯度和高预示效率的单光子。使用 10 米单模光纤建立了连接，使得量子传送可以从一个芯片到另一个芯片。右侧的子面板是微环谐振器结构和传送接收器。 HOM，Hong–Ou–Mandel 效应；BSM，贝尔态测量；CC，经典通道；QC，量子通道；FBG，光纤布拉格光栅；IM，强度调制器；FM，法拉第镜；MZI，马赫-曾德干涉仪；BS，光束分 splitter；PBS，偏振光束分 splitter；HWP，半波片；LD，激光二极管；PDs，光电二极管；PPLN，周期性极化铌酸锂；DM，二色镜；FP，法布里-珀罗腔；FPGA，现场可编程门阵列；VEDL，可变电子延迟线；DWDM，密集波长分复用器；AWG，任意波形发生器；Si-APD，硅雪崩光电二极管；SPD，单光子探测器；SNSPD，超导纳米线单光子探测器；CMOS，相机；SHG，二次谐波生成；SPDC，自发参数下转换。面板 a 摘自参考文献 18，Springer Nature Ltd；面板 b 摘自参考文献 16，Springer Nature Ltd；面板 c 摘自参考文献 27，Springer Nature Ltd。

Full size image

In the Hefei experiment¹⁷, the transmitted state was encoded with true single photons⁷⁷ at the telecom wavelength, and an active feed-forward scheme was used to increase the BSM success probability to 50%. This was the first time quantum teleportation was achieved with independent quantum sources, previous entanglement distribution and active feed-forward operations simultaneously in a long-distance optical fibre network. In this experiment, the error signal derived from the photon arrival time in the feedback loop was used to reduce the total arrival time jitter of photons from the remote nodes to 6 ps, which is negligible compared with the photon coherence time of 110 ps.
在合肥实验中，传输状态使用电信波长的单光子进行编码，并采用主动反馈方案将贝尔态测量（BSM）的成功概率提高到 50%。这是首次在长距离光纤网络中实现量子传送，同时使用独立的量子源、先前的纠缠分发和主动反馈操作。在该实验中，反馈回路中由光子到达时间产生的误差信号被用来将来自远程节点的光子总到达时间抖动降低到 6 皮秒，这与 110 皮秒的光子相干时间相比是微不足道的。

In the Calgary experiment¹⁸ (Fig. 3a), the transmitted state was not encoded with single photons, but with an attenuated laser beam, a method that can be applied to a decoy state protocol developed through quantum cryptography¹⁷³. This was different from the Hefei experiment in that it used nondegenerate entangled photon sources with wavelengths of 1550 nm and 795 nm. The state was teleported from 1550-nm photons to 795-nm photons, which permits more efficient and robust photon detectors to be used around Bob. These different settings made this experiment achieve a higher transmission rate of 12 clicks per minute.
在卡尔加里实验中（图 3a），传输状态不是通过单光子编码，而是通过衰减激光束编码，这种方法可以应用于通过量子密码学开发的诱骗态协议。这与合肥实验不同，因为它使用了波长为 1550 nm 和 795 nm 的非简并纠缠光子源。状态从 1550 nm 光子传送到 795 nm 光子，这允许在鲍勃周围使用更高效和更稳健的光子探测器。这些不同的设置使得该实验实现了每分钟 12 次的更高传输速率。

The aforementioned two experiments show that a quantum network spanning cities is realistic. The entangled photon sources in these experiments were generated through spontaneous parametric down-conversion (SPDC)⁷⁷. The random nature of this process limits the generation rate for multiphoton states, but more recently, great progress has been made in developing on-demand solid-state quantum emitters. Quantum teleportation using solid-state emitters has been demonstrated successfully^{174,175,176,177}, providing an alternative high-speed solution to SPDC. The development of superconducting detectors has significantly improved photon detection efficiency in the communication band, thus increasing the teleportation distance to over 100 km^178,179,180. One experiment¹⁸¹ used a high-performance time bin entangled quantum light source with a single-fibre-pigtailed periodically poled lithium niobate (PPLN) waveguide to achieve high-speed quantum teleportation along a 64-km-long fibre channel at a rate of 7.1 Hz. CV quantum teleportation through a 6-km optical fibre has been also realized in the laboratory¹⁸². Some experiments have also verified that decoherence in quantum teleportation can be effectively reduced by regulating the channel noise^183,184.
上述两个实验表明，跨城市的量子网络是现实的。这些实验中的纠缠光子源是通过自发参量下转换（SPDC）生成的。该过程的随机性限制了多光子态的生成速率，但最近在开发按需固态量子发射器方面取得了重大进展。使用固态发射器的量子隐形传态已成功演示，为 SPDC 提供了一种替代的高速解决方案。超导探测器的发展显著提高了通信波段的光子探测效率，从而将隐形传态距离增加到超过 100 公里。一项实验使用高性能时间窗纠缠量子光源与单光纤尾纤周期性极化铌酸锂（PPLN）波导，实现在 64 公里长的光纤通道中以 7.1 Hz 的速率进行高速量子隐形传态。通过 6 公里光纤的连续变量量子隐形传态也在实验室中实现。 一些实验还验证了通过调节通道噪声可以有效减少量子传输中的去相干现象 183,184。

Free space 自由空间

Owing to the unavoidable photon loss of the distribution channel, the transmission distance of quantum teleportation using optical fibres^178,179 and ground-free space^185,186 channels was limited to about 100 km in previous studies. Realizing a ‘quantum internet’¹⁸⁷ on a global scale requires greatly expanding the scope of quantum teleportation.
由于分配通道不可避免的光子损失，使用光纤和地面自由空间通道的量子传送在以往研究中传输距离限制在约 100 公里。实现全球范围的“量子互联网”需要大幅扩展量子传送的范围。

One method is to use quantum repeaters^164,165,166 to continuously complete quantum entanglement swapping to extend the distance of photon entanglement over a long distance. However, quantum repeaters need quantum memory¹⁵⁶ and quantum interface¹⁸⁸ technologies with excellent performance, and these are still in the laboratory verification stage.
一种方法是使用量子中继器 164,165,166，持续完成量子纠缠交换，以延长光子纠缠的距离。然而，量子中继器需要具有优异性能的量子存储 156 和量子接口 188 技术，而这些仍处于实验室验证阶段。

Another promising way is to use satellite platforms and space-based links to connect remote modes on Earth. In this case, most of the photon propagation paths are in empty space, which greatly reduces channel loss and decoherence. Many satellite projects have been proposed for quantum communications, such as the Micius satellite¹⁸⁹, the Quantum Encryption and Science Satellite (QEYSSat) project in Canada¹⁹⁰ and the CubeSat Quantum Communications Mission (CQuCoM), undertaken by a joint research team¹⁹¹.
另一种有前景的方法是利用卫星平台和基于空间的链接来连接地球上的远程模式。在这种情况下，大多数光子传播路径位于空旷的空间中，这大大减少了信道损耗和去相干。许多卫星项目已被提议用于量子通信，例如墨子卫星、加拿大的量子加密与科学卫星（QEYSSat）项目以及由一个联合研究团队承担的立方卫星量子通信任务（CQuCoM）。

In 2017, quantum teleportation was achieved from a ground observatory station in Ngari to the Micius satellite¹⁶ (Fig. 3b). The ultra-bright four-photon sources had a wavelength of 780 nm¹⁹², the counting rates were 5.7 × 10⁵ s⁻¹ for the teleported photons and 1 × 10⁶  s⁻¹ for the frequency-uncorrelated entangled photon pairs and the fidelity was 0.933. The experiment optimized the efficiency of entanglement distribution and overcame atmospheric turbulence in the uplink by using narrow beam divergence, high bandwidth, high-precision acquisition, pointing and tracking techniques (Fig. 3b). The average teleportation fidelity for the six input states was F = 80 ± 1% > 2/3. As for CV systems, this scheme can also perform remote quantum teleportation via satellite connection¹⁹³. More complex space-to-ground quantum teleportation is expected to be realized soon and to have a key role in the future space–ground quantum internet¹⁹⁴.
在 2017 年，从那曲的地面观测站实现了量子传送到墨子卫星 16（图 3b）。超亮的四光子源波长为 780 纳米 192，传送光子的计数率为 5.7 × 10^5 s−1，频率无关的纠缠光子对的计数率为 1 × 10^6 s−1，保真度为 0.933。该实验优化了纠缠分发的效率，并通过使用窄光束发散、高带宽、高精度采集、指向和跟踪技术克服了上行链路中的大气湍流（图 3b）。六个输入态的平均传送保真度为 F = 80 ± 1% > 2/3。至于连续变量系统，该方案也可以通过卫星连接进行远程量子传送 193。预计更复杂的空间到地面的量子传送将很快实现，并在未来的空间-地面量子互联网中发挥关键作用 194。

Integrated chips 集成电路芯片

Integrated quantum information processors provide an excellent opportunity for developing quantum communication with superior performance because of their high stability and scalability. Integrated quantum photonics^195,196,197 has made rapid progress in recent years, which has enabled high-quality generation, processing and detection of multiphoton states^198,199.
集成量子信息处理器为开发具有优越性能的量子通信提供了极好的机会，因为它们具有高稳定性和可扩展性。近年来，集成量子光子学取得了快速进展，这使得多光子态的高质量生成、处理和检测成为可能。

In 2014, quantum teleportation was demonstrated in an ultraviolet-written silicon photonic chip²⁰⁰. However, a major challenge facing this setup is implementing sufficiently high-quality multiphoton sources and multiqubit operators in a single integrated system.
在 2014 年，量子传送在紫外线写入的硅光子芯片上得以实现。然而，这一设置面临的主要挑战是如何在单一集成系统中实现足够高质量的多光子源和多量子比特操作。

In 2020, another group realized fully integrated interchip quantum teleportation²⁷. The design of on-chip microring resonators (Fig. 3c) achieved a spectral purity of 92% and a two-photon interference of 90.99 ± 3.91%. The heralding efficiency after the resonators was measured to be 50%, which means that the signal photon of a photon pair can escape the resonator with a probability of 50%. The transmitter and receiver were coherently linked by a 10-m single-mode fibre, enabling quantum teleportation between chips. The teleported states were recovered on the receiver chip with an average fidelity of F = 88.5 ± 3.7%. This chip was also used to prepare three-photon and four-photon Greenberger–Horne–Zeilinge states with fidelities of F₃ = 73.5 ± 1.7% and F₄ = 68.3 ± 1.4%.
在 2020 年，另一组实现了完全集成的芯片间量子传送。片上微环谐振器的设计达到了 92%的光谱纯度和 90.99 ± 3.91%的双光子干涉。谐振器后的预示效率测量为 50%，这意味着光子对的信号光子以 50%的概率可以逃离谐振器。发射器和接收器通过 10 米的单模光纤相干连接，实现了芯片间的量子传送。传送的状态在接收芯片上以平均保真度 F = 88.5 ± 3.7%恢复。该芯片还用于制备三光子和四光子格林伯格-霍恩-齐林格态，保真度分别为 F3 = 73.5 ± 1.7%和 F4 = 68.3 ± 1.4%。

Scalable integrated optical quantum chips permit to implement high-dimensional quantum information processing tasks^80,201. In 2022, experimenters applied unsupervised machine learning to train an on-chip autoencoder to encode 3D quantum states onto 2D quantum teleportation, observing 3D teleportation with a fidelity of 0.894 (ref. ²⁰²). With the development of integrated quantum chips, an on-chip high-dimensional quantum teleportation is expected to be realized, which contains high-dimensional quantum-state transmission and high-dimensional BSM.
可扩展的集成光学量子芯片允许实现高维量子信息处理任务。2022 年，实验者应用无监督机器学习训练了一个片上自编码器，将 3D 量子态编码到 2D 量子传送中，观察到 3D 传送的保真度为 0.894（参考文献 202）。随着集成量子芯片的发展，预计将实现片上高维量子传送，其中包含高维量子态传输和高维贝尔态测量。

Quantum teleportation with an integrated quantum memory has also been investigated. For example, high-frequency nano-optical systems²⁰³ can be used for quantum memory in optical communication and are therefore promising as nodes in a future quantum network. In 2021, a polarization-encoded optical input state was teleported into the joint state of a pair of integrated nanomechanical resonators²⁰⁴. The mechanical coherence lifetimes of the two integrated nanomechanical resonators were $T_1^{(A)}=1.3\mu \mathrm{s}$ and $T_1^{(B)}=1.9\mu \mathrm{s}$, which should be long enough to store the teleported state until it is read. In 2022, an experiment demonstrated long-distance quantum teleportation from a photonic qubit to a 17.5 μs rare-earth crystal quantum memory through a 1-km fibre channel²⁰⁵. Integrated quantum teleportation will be realized in the future by combining integrated solid-state quantum memory^206,207,208 with simultaneously integrated entangled photon sources and interfaces.

Gate quantum teleportation (Fig. 1c) is an important variant of quantum teleportation that distributes local gate operations between spatially separated particles (Fig. 4a).
门量子传输（图 1c）是量子传输的重要变体，它在空间上分离的粒子之间分配局部门操作（图 4a）。

a, Concept of distributed quantum computing and gate teleportation with superconducting qubits. The left side illustrates distributed quantum computing in a quantum network. Divide the particles in each node of quantum computing into data qubit (qubits) (red) and communication qubit (qubits) (blue). Each node is connected by entanglement (purple), and different nodes perform synchronous quantum computing through quantum gate teleportation. The circuit diagram for gate quantum teleportation is shown in the middle; it consists of four steps: first, entanglement of two communication qubits (blue line); second, controlled gate operation of data qubit (red line) to communication qubit; third, perform X and Z Pauli measurements on two communication qubits separately; fourth, perform X and Z operations on the data qubits on the basis of the measurement results. The right side shows quantum gate teleportation with superconducting qubits²². The gate teleportation consists of a logical qubit defined as a coaxial quarter wavelength (λ/4) 3D cavity (data qubit (red)) and an entanglement qubit defined as a Y-shaped transmon qubit (communication qubit (blue)) . b, Setup for quantum gate teleportation with trapped ions²³. The blue dots represent ⁹Be⁺, and the red dots represent ²⁵Mg⁺ ions. A–F shows ions trapped in a segmented linear Pauli trap, which can be moved between different regions of the trap. Therefore, the experimenters can simplify the C-NOT gate by using only one laser interaction zone (LIZ) and transporting the separated qubits to this location. The experiment demonstrates a deterministic teleported C-NOT between two ⁹Be⁺ ions using a shared engaged pair of ²⁵Mg⁺ ions. c, Quantum-state teleportation in cavity quantum electrodynamics (QED)²²⁷. Two cavity QED setups are connected with a 60-m-long optical fibre. This is different from the previous quantum teleportation protocols because a single photon is used as the medium, and the QED cavity on both sides completes the C-NOT gate operation in turn with the single photon. Then the experimenter performs projection measurements on the single photon and on the QED cavity of Alice, and the measurement results are reported to Bob through the classical channel to complete the quantum teleportation. This protocol can achieve C-NOT gate operation of two remotely located QED cavity nodes with slight changes²⁴. A/D, antidiagonal/diagonal polarization; NPBS, non-polarized beam splitter. Panel a reproduced from ref. ²², Springer Nature Ltd; panel b reproduced from ref. ²³, AAAS; panel c reproduced from ref. ²²⁷ under a Creative Commons licence CC BY 4.0.
分布式量子计算和超导量子比特的门传送概念。左侧展示了量子网络中的分布式量子计算。将每个量子计算节点中的粒子分为数据量子比特（红色）和通信量子比特（蓝色）。每个节点通过纠缠（紫色）连接，不同节点通过量子门传送进行同步量子计算。中间的电路图展示了门量子传送的四个步骤：第一，两个通信量子比特的纠缠（蓝线）；第二，数据量子比特（红线）对通信量子比特的受控门操作；第三，分别对两个通信量子比特进行 X 和 Z 泡利测量；第四，根据测量结果对数据量子比特进行 X 和 Z 操作。右侧展示了超导量子比特的量子门传送。门传送由一个定义为同轴四分之一波长（λ/4）的三维腔体的逻辑量子比特（数据量子比特（红色））和一个定义为 Y 形 transmon 量子比特的纠缠量子比特（通信量子比特（蓝色））组成。 b，带有捕获离子的量子门传送设置 23。蓝点代表 9Be+，红点代表 25Mg+离子。A-F 显示了被困在分段线性保利陷阱中的离子，这些离子可以在陷阱的不同区域之间移动。因此，实验者可以通过仅使用一个激光相互作用区（LIZ）来简化 C-NOT 门，并将分离的量子比特传送到该位置。该实验演示了在两个 9Be+离子之间使用一对共享的 25Mg+离子进行确定性传送的 C-NOT。c，腔量子电动力学（QED）中的量子态传送 227。两个腔 QED 设置通过一根 60 米长的光纤连接。这与之前的量子传送协议不同，因为使用单个光子作为媒介，且两侧的 QED 腔依次完成 C-NOT 门操作。然后，实验者对单个光子和爱丽丝的 QED 腔进行投影测量，测量结果通过经典通道报告给鲍勃，以完成量子传送。 该协议可以通过稍微的改动实现两个远程位置的量子电动力学腔节点的 C-NOT 门操作。A/D，反对角/对角偏振；NPBS，非偏振光束分裂器。面板 a 转载自参考文献 22，Springer Nature Ltd；面板 b 转载自参考文献 23，AAAS；面板 c 转载自参考文献 227，依据创意共享许可证 CC BY 4.0。

Full size image

In 1999, a theoretical proposal suggested that a universal quantum computer could be built using only quantum teleportation, local operations and classical communication³². More importantly, teleportation-based quantum computing can effectively block propagating errors so that fault-tolerant quantum computing can be expanded on a large scale.
在 1999 年，一项理论提议表明，可以仅使用量子传送、局部操作和经典通信来构建一个通用量子计算机。更重要的是，基于传送的量子计算能够有效阻止传播错误，从而使容错量子计算能够大规模扩展。

Gate teleportation is also the core of quantum computing based on cluster states^49,209 and other kinds of measurement-based quantum computing²¹⁰. In this model, gate operations can be distributed through quantum gate teleportation among different particles that come from multipartite entangled states.
门传送也是基于簇态的量子计算 49,209 和其他类型的基于测量的量子计算 210 的核心。在该模型中，门操作可以通过量子门传送在来自多体纠缠态的不同粒子之间分配。

Both entanglement distribution and state transfer via teleportation are necessary in quantum networks⁶⁰. In a quantum network^19,187, quantum teleportation is the core technology for remote connection of quantum computers, to achieve synchronous distributed computing of different nodes^20,21,211.
在量子网络中，纠缠分布和通过传送态的状态转移都是必要的。量子传送是量子网络中远程连接量子计算机的核心技术，以实现不同节点的同步分布式计算。

Quantum gates 量子门

Quantum gate teleportation was first demonstrated in a photonic system^212,213 and could be applied in linear optical quantum computing theory²¹⁴. Quantum gate teleportation has also been implemented with superconducting qubits²², trapped ions²³, QED cavities²⁴ and quantum dot spin qubits^215,216. These systems have excellent scalability and are candidates for future large-scale fault-tolerant quantum computers^217,218.
量子门传送首次在光子系统中得到验证 212,213，并可应用于线性光学量子计算理论 214。量子门传送还已在超导量子比特 22、被捕获离子 23、量子电动力学腔 24 和量子点自旋量子比特 215,216 中实现。这些系统具有优良的可扩展性，是未来大规模容错量子计算机的候选者 217,218。

In 2018, gate teleportation was demonstrated using a superconducting cavity²¹⁹ coupled to a superconducting circuit qubit²². This setup had a record quantum lifetime (approximately 1 ms) and sub-microsecond feed-forward controls (Fig. 4a). In addition, the experiment used an error-correcting code that uses the bosonic nature of the cavity. This is more robust than standard qubits. With error corrections, the process fidelity of the gate teleportation was increased from 68 ± 2% to 79 ± 2%. A propagating microwave is an important carrier of the superconducting circuit interface. In another experiment, a propagating coherent microwave state was teleported over a distance of d = 0.42 m in the laboratory²²⁰. Superconducting qubit entanglement has achieved a 10-m distribution^{221,222,223,224}. These advances lay the foundation for quantum gate teleportation of remotely separated superconducting qubits.
在 2018 年，使用耦合到超导电路量子比特的超导腔体演示了门传送。这一设置具有创纪录的量子寿命（约 1 毫秒）和亚微秒的前馈控制（图 4a）。此外，实验使用了一种利用腔体玻色子特性的纠错码。这比标准量子比特更为稳健。通过纠错，门传送的过程保真度从 68 ± 2%提高到 79 ± 2%。传播的微波是超导电路接口的重要载体。在另一个实验中，传播的相干微波态在实验室中传送了 d = 0.42 米的距离。超导量子比特纠缠已实现 10 米的分布。这些进展为远程分离的超导量子比特的量子门传送奠定了基础。

In 2019, an entangled pair of ²⁵Mg⁺ ions was used to verify deterministic gate teleportation between two ⁹Be⁺ ions²³ (Fig. 4b). From the measurement outcomes, the experimenters determined the quantum process by maximum likelihood estimation and inferred a 95% confidence interval of (0.845, 0.872) for the entanglement fidelity with respect to an ideal C-NOT gate. The interface between a photon and trapped ion has been used to entangle two ion traps 230 m apart²²⁵. In the future, similar technologies can be used to achieve ion-based remotely distributed quantum computing in quantum networks.
在 2019 年，一对纠缠的 25Mg+离子被用来验证两个 9Be+离子之间的确定性门传送（图 4b）。通过测量结果，实验者通过最大似然估计确定了量子过程，并推断出相对于理想 C-NOT 门的纠缠保真度的 95%置信区间为(0.845, 0.872)。光子与被捕获离子之间的接口已被用来纠缠相距 230 米的两个离子阱。未来，类似的技术可以用于在量子网络中实现基于离子的远程分布式量子计算。

In 2021, quantum teleportation was achieved between two QED cavities²²⁶ connected through a 60-m optical fibre²²⁷ (Fig. 4c). This approach demonstrates a new teleportation protocol that, in principle, allows for unconditional teleportation without the need for the commonly used pre-shared entanglement resource. This scheme requires two C-NOT operations between the QED cavity nodes and the auxiliary photon. Using this new quantum teleportation scheme, the experimenters realized a remote C-NOT operation²⁴ between QED cavity nodes with a spacing of 60 m. The experiment achieved an average overlap fidelity of 76.6 ± 1.0% with ideal Bell states. In this scheme, only one single-photon transmission and measurement is used for teleportation, effectively reducing the consumption of entangled photon pairs, and providing a new solution for large-scale and long-distance quantum networks.
在 2021 年，量子传送在两个通过 60 米光纤连接的量子电动力学腔体之间实现了。这种方法展示了一种新的传送协议，原则上允许无条件传送，而无需常用的预共享纠缠资源。该方案需要在量子电动力学腔体节点和辅助光子之间进行两个 C-NOT 操作。利用这一新的量子传送方案，实验者实现了在 60 米间距的量子电动力学腔体节点之间的远程 C-NOT 操作。实验达到了与理想贝尔态的平均重叠保真度为 76.6 ± 1.0%。在该方案中，仅使用一次单光子传输和测量进行传送，有效减少了纠缠光子对的消耗，为大规模和长距离量子网络提供了一种新解决方案。

Individual electron spins in semiconductor quantum dots are a candidate platform for quantum computing owing to their long correlation time and scalability²²⁸. Two experiments used quantum dot spin qubits to realize quantum-state teleportation, entanglement swapping and gate teleportation^215,216, which can be used to create and manipulate long-range states and correct errors in quantum dot spin qubits.
半导体量子点中的单个电子自旋由于其长相关时间和可扩展性，成为量子计算的候选平台 228。两个实验利用量子点自旋量子比特实现了量子态传送、纠缠交换和门传送 215,216，这可以用于创建和操控长程态以及纠正量子点自旋量子比特中的错误。

Multiple nodes 多个节点

In future quantum networks, qubits implemented in atomic²²⁷, trapped-ion²³ and various other systems^22,229 will form network nodes for quantum computing, quantum sensing and other applications. Through flying qubits, such as microwave photons, quantum teleportation will be used to send quantum information between these quantum nodes. Connecting multiple nodes or non-neighbouring nodes becomes a major challenge as the number of nodes increases. In recent years, entanglement between three cold atomic ensembles²³⁰ or three independent NV centre spins²³¹ has been achieved by optical interference postselection or local spin entanglement. In these studies, the three nodes were neighbours, and the non-neighbouring nodes were connected by quantum teleportation.
在未来的量子网络中，实施在原子、被捕获离子和各种其他系统中的量子比特将形成量子计算、量子传感和其他应用的网络节点。通过飞行量子比特，如微波光子，量子隐形传态将用于在这些量子节点之间发送量子信息。随着节点数量的增加，连接多个节点或非邻近节点成为一个主要挑战。近年来，通过光学干涉后选择或局部自旋纠缠，实现了三组冷原子集体或三个独立的 NV 中心自旋之间的纠缠。在这些研究中，三个节点是邻近的，非邻近节点通过量子隐形传态连接。

In 2022, an experiment showed quantum teleportation between NV qubit nodes without direct connection through quantum channels²⁸. The experimenters used NV qubits as the three nodes to construct a quantum network and used NV electronic spin as the communication qubit and a nearby ¹³C nuclear spin as a memory qubit. First, an entanglement of non-neighbouring nodes was established through quantum entanglement swapping, and then quantum teleportation was conducted between two nodes. The average teleported state fidelity was 70.2 ± 1.1% at a rate of 0.5 per minute. Compared with previous efforts to entangle three nodes^230,231, this experiment clearly demonstrated multinode quantum repeaters^164,165,166 which is an important functional tool in quantum networks. The advantage of memory qubits is that they can be used to read out and protect qubit information while generating entanglement and, at the same time, achieve the real-time rejection of false remaining signals. Quantum teleportation between multiple nodes should also be realized in other solid-state^160,161 or atomic systems^6,158 in the near future.
在 2022 年，一项实验显示了在没有通过量子通道直接连接的情况下，NV 量子比特节点之间的量子传送。实验者使用 NV 量子比特作为三个节点构建量子网络，并使用 NV 电子自旋作为通信量子比特，附近的 13C 核自旋作为存储量子比特。首先，通过量子纠缠交换建立了非邻近节点的纠缠，然后在两个节点之间进行了量子传送。平均传送态保真度为 70.2 ± 1.1%，传送速率为每分钟 0.5 次。与之前对三个节点进行纠缠的努力相比，这项实验清楚地展示了多节点量子中继，这是量子网络中的一个重要功能工具。存储量子比特的优势在于它们可以在生成纠缠的同时读取和保护量子比特信息，并实现对虚假剩余信号的实时拒绝。多节点之间的量子传送在不久的将来也应在其他固态或原子系统中实现。

Quantum error correction 量子错误纠正

Quantum error correction is essential for reliable quantum information processing on a large scale^217,218. Quantum teleportation is an important means to encode physical qubits into logical qubits²³².
量子错误纠正对于大规模可靠的量子信息处理至关重要 217,218。量子隐形传态是将物理量子比特编码为逻辑量子比特的重要手段 232。

In 2021, two experiments demonstrated the teleportation of physical qubits to fault-tolerant logical qubits. In one experiment²³³, a photonic polarization qubit was teleported to a logical qubit of the nine-qubit Shor code²³⁴, and the nine qubits were encoded in three DoFs (polarization, path and OAM) of three photons. A bit flip of one of the nine physical qubits encoding the logical qubit resulted in a change in the error syndrome measurements and thus could be excluded. After correction, the average fidelity of the three teleported states was F = 78.6 ± 1.7%, well exceeding the classical bound of two-thirds.
在 2021 年，两项实验展示了物理量子比特到容错逻辑量子比特的传送。在其中一项实验中，一个光子极化量子比特被传送到九量子比特 Shor 编码的逻辑量子比特中，这九个量子比特被编码在三个光子的三个自由度（极化、路径和轨道角动量）中。对编码逻辑量子比特的九个物理量子比特之一进行比特翻转导致错误综合测量的变化，因此可以被排除。在纠正后，三种传送状态的平均保真度为 F = 78.6 ± 1.7%，远超经典界限的三分之二。

The other experiment²³⁵ simulated the teleportation of a physical superconducting qubit to a logical code qubit (the Majorana zero mode qubit²³⁶). The Majorana code is a quantum error-detecting code for phase-flip errors that is used to improve the average fidelity of teleportation for six states from 70.76 ± 0.35% to 84.60 ± 0.11%, well beyond the classical bound in either case.
另一个实验模拟了物理超导量子比特到逻辑编码量子比特（马约拉纳零模量子比特）的传送。马约拉纳编码是一种用于相位翻转错误的量子错误检测编码，用于将六个状态的平均保真度从 70.76 ± 0.35%提高到 84.60 ± 0.11%，在任何情况下都远超经典界限。

Since 2015, developments in quantum teleportation technology have mainly focused on transitioning from simple to complex quantum states and from proof-of-principle demonstrations to practical applications. Table 1 summarizes the performance of various systems in quantum teleportation. Flying photonic qubits are often used in long-distance quantum communication media, and atomic and solid-state systems are often used in quantum computing nodes. The two can be combined to construct quantum networks using quantum teleportation¹⁹.
自 2015 年以来，量子传输技术的发展主要集中在从简单量子态到复杂量子态的过渡，以及从原理验证演示到实际应用的转变。表 1 总结了各种量子传输系统的性能。飞行光子量子比特通常用于长距离量子通信媒介，而原子和固态系统则常用于量子计算节点。这两者可以结合起来，利用量子传输构建量子网络。

Developments in the teleportation of complex quantum states have mainly focused on photonic systems, the challenges being the preparation of entanglement¹² and the BSM in complex quantum states¹²⁰. In photonic systems, BSM with multiple DoFs or high dimensions in linear optics requires auxiliary particles^{13,14,15,88,89,92,237} or nonlinear processes^124,238. The randomness of SPDC^77,239 and the inefficient nonlinear process²⁴⁰ limit the success probability of quantum teleportation. Quantum teleportation via a deterministic photon source^49,241,242 and highly efficient on-chip nonlinearity²⁴³ may be the key to improving this. These new technologies will also greatly increase the transmission rate of quantum teleportation based on urban fibre-optic networks and satellites. High-dimensional entanglement of trapped-ion^108,244, NV centres¹⁰⁹ and superconducting qubits^107,244 have also been reported. Deterministic BSM can be performed in these systems without auxiliary particles^28,224,245. These advances suggest that quantum teleportation of complex states will be realized in these systems in the near future.
复杂量子态的传送发展主要集中在光子系统上，面临的挑战是纠缠的制备和复杂量子态中的贝尔态测量。在光子系统中，线性光学中具有多个自由度或高维度的贝尔态测量需要辅助粒子或非线性过程。自发参量下转换的随机性和低效的非线性过程限制了量子传送的成功概率。通过确定性光子源和高效的片上非线性实现的量子传送可能是改善这一点的关键。这些新技术还将大大提高基于城市光纤网络和卫星的量子传送传输速率。被捕获离子的高维纠缠、氮-空位中心和超导量子比特也已被报道。这些系统中可以在没有辅助粒子的情况下进行确定性贝尔态测量。这些进展表明，复杂态的量子传送将在不久的将来在这些系统中实现。

Quantum teleportation can be used in quantum communication to overcome the distance limit of direct transmission of quantum states. A variation of quantum teleportation is quantum entanglement swapping, which is the core technology in quantum repeaters²⁴⁶. A quantum memory is essential in synchronizing entanglement swapping of all nodes to maximize the quantum information transmission rate^156,164. Quantum swapping can be enhanced by developing large-bandwidth, long-life and high-fidelity solid-state memories^160,161 and atomic memories^247,248. The method of increasing the success probability of BSM by adding auxiliary photons^88,89,92 or using error correction codes^90,91 is expected to be applied in quantum teleportation in the near future to increase the quantum communication rate of each node. We can also expect quantum repeaters to achieve quantum communication beyond the rate of direct optical fibre communication in the near future.
量子隐形传态可以用于量子通信，以克服量子态直接传输的距离限制。量子隐形传态的一种变体是量子纠缠交换，这是量子中继的核心技术。量子存储器在同步所有节点的纠缠交换中至关重要，以最大化量子信息传输速率。通过开发大带宽、长寿命和高保真度的固态存储器和原子存储器，可以增强量子交换。通过添加辅助光子或使用纠错码来提高贝尔态测量成功概率的方法预计将在不久的将来应用于量子隐形传态，以提高每个节点的量子通信速率。我们也可以期待量子中继在不久的将来实现超越直接光纤通信速率的量子通信。

Developments in the application of quantum teleportation in distributed quantum computing have focused mainly on solid-state systems (superconducting qubits and NV centres) and atomic systems (QED cavities and ion traps). Although a quantum advantage has been demonstrated in quantum computing^9,249, many quantum algorithms (including Shor’s and Grover’s algorithms) require large numbers of qubits and long-range interactions. Quantum teleportation is expected to solve the problem of long-range interactions between different particles^20,32,250. Arrays of neutral atom^251,252, integrated magneto-optical traps²⁵³ and ion trap chips²⁵⁴ have effective scaling potential, which is the dawn of large-scale distributed quantum computing based on quantum teleportation^20,21.
量子传输在分布式量子计算中的应用发展主要集中在固态系统（超导量子比特和氮空位中心）和原子系统（量子电动力学腔和离子阱）。尽管在量子计算中已经证明了量子优势，但许多量子算法（包括肖尔算法和格罗弗算法）需要大量的量子比特和长程相互作用。量子传输有望解决不同粒子之间的长程相互作用问题。中性原子阵列、集成磁光阱和离子阱芯片具有有效的扩展潜力，这标志着基于量子传输的大规模分布式量子计算的曙光。

Quantum teleportation will be necessary for quantum networks with photons interfacing with solid-state or atomic systems as the computing nodes¹⁹. Two-node gate teleportation has been achieved over a distance of 60 m, but there are still major challenges before achieving a distance on the scale of kilometres or more. One of these challenges is due to photons emitted or absorbed by atomic or solid-state systems often being incompatible with existing fibre-optic networks. Advances in areas such as on-chip frequency conversion technology²⁴³, non-degenerate entanglement sources²⁵⁵, hybrid quantum teleportation⁷⁴ and hollow-core optical fibres²⁵⁶ should help overcome this issue.
量子隐形传态对于与固态或原子系统作为计算节点的光子接口的量子网络是必要的。两节点门传态已在 60 米的距离上实现，但在实现公里级或更长距离之前仍面临重大挑战。其中一个挑战是，原子或固态系统发射或吸收的光子通常与现有的光纤网络不兼容。在芯片频率转换技术、非简并纠缠源、混合量子隐形传态和空心光纤等领域的进展应有助于克服这一问题。